Confidence Intervals for the Difference between Two Means

Often when comparing two populations there is a need to see if there is a significant difference between the means of the populations. e.g. Comparing the average weights of fruit from two different trees.

In the same way that the Central Limit Theorem allowed us to calculate the mean and standard deviation for the sample means and sample proportions, we can do the same for the difference between two means.

Thus confidence intervals for the difference between two means can be calculated to a desired degree of confidence, provided the two samples are independent.

Degree of Confidence

The degree of confidence is given as a percentage probability changed into a z-score, using the standard normal distribution tables. Check out where the z-scores are found in the tables for the following degrees of confidence.

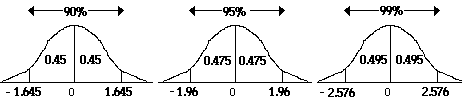
Y12_Confidence_Intervals_for_the_Difference_between_Two_Means_01.gif

Any degree of confidence can be found in this manner. e.g. For an 80% level of confidence look up 0.40 in the tables.

Confidence Interval for the Difference between Two Means

The confidence interval, based on the Central Limit Theorem, for the difference between two means μ1 and μ2 is given by:

Y12_Confidence_Intervals_for_the_Difference_between_Two_Means_02.gif

where n1 and n2 are the sizes of the two samples and 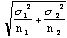Y12_Confidence_Intervals_for_the_Difference_between_Two_Means_03.gif is the standard deviation of the sampling distribution of the difference between two sample means.

The value of z will depend upon the degree of confidence required. The z values for 90, 95 and 99% are shown above.

If the population standard deviations (σ1 and σ 2 ) are not known the sample standard deviations can be used as an estimate.

Example

A sample of 60 students from schools in the North Island has a mean weight of 55 kg with a standard deviation of 5 kg. A sample of 50 students from schools in the South Island has a mean of 53 kg and a standard deviation of 7 kg. Find a 99% confidence interval for the difference between the means of the population of students in the two islands.

Let n1 = 60 and n2 = 50

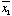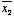Y12_Confidence_Intervals_for_the_Difference_between_Two_Means_04.gif = 55 and Y12_Confidence_Intervals_for_the_Difference_between_Two_Means_05.gif = 53
Use the sample standard deviations s1 = 5 and s2 = 7
z = 2.576 for a 99% level of confidence.

Using 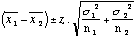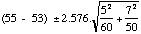Y12_Confidence_Intervals_for_the_Difference_between_Two_Means_06.gif = Y12_Confidence_Intervals_for_the_Difference_between_Two_Means_07.gif

The 99% confidence interval is (-1.04, 5.04)

This can be written as -1.04 < μ1 and μ2 < 5.04.

As this interval includes 0, this means that the two populations could have the same mean.