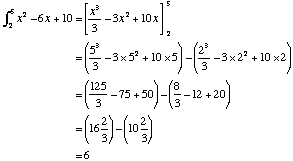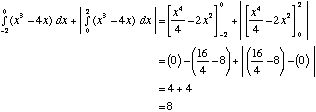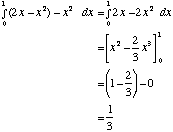Differentiation gives the gradient of a curve at a particular point.
Integration has a wide range of applications incuding finding volumes, heat, mass and areas.
In earlier topics, we estimated the area under curves using the Trapezium Rule and Simpson's Rule, we now study a more precise method.
The definite integral  gives the area shaded on the right. |
 |
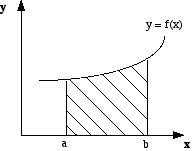Area between Curve and x-axis
To find the area between a curve, two x-values and the x-axis, as shaded in the example on the diagram below, evaluate the definite integral between those x-values.
|
Example Find the shaded area between the curve f(x) = x2-6x + 10 , the lines x = 2 and x = 5 and the x-axis. Evaluate the definite integral:
The shaded area is 6 square units.
|
 |
(x2 − 6x + 10) dx
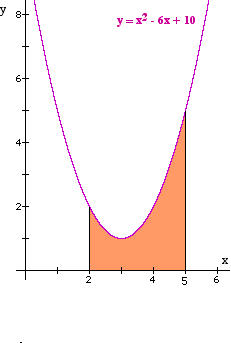It is advisable, although not essential to draw a diagram before carrying out the integration.
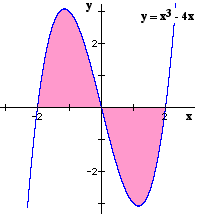
Area below x-axis
Sometimes, when the curve crosses the x-axis, part of the area may be above the x-axis and part below the x-axis.
When this happens evaluate each area separately, treating both as positive, and then add them together.
The absolute (positive) signs indicate this in the example below.
|
Example Find the shaded area: Evaluate:
|
 |
Area between Two Curves
If the area between two curves is required then the following rule works well.
Area between two functions = ∫ (top function − lower function) dx
|
Example Find the shaded area between the functions Evaluate:
|
 |
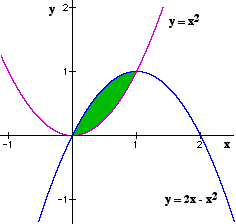