|
Problem Solving |
|
Answer 1. $64.26 2. The 24th house 3. 10,000 4. 528 pies 5. 8 dozen gingerbread men 6. 106 hours
|
|
|
Problem Solving |
|
|
What fraction of the hexagon is shaded?
Answer 2/3 of the hexagon is shaded.
|
|
|
Problem Solving |
|
|
Percentages a. Which is greater 35% of 45 or 45% of 35? b. Which is greater? An increase of 10% followed by a decrease of 10% or a decrease of 20% followed by an increase of 20%? c. At a High School, 90% of the students take mathematics, 85% take science and 80% take geography. What percentage, at least, must take all three subjects?
Answer a. The amounts are equal. b. Increase of 10% followed by a decrease of 10%
|
|
|
Problem Solving |
|
|
What number comes next? 7, 9, 16, 25, 41, 66
Answer 107
|
|
|
Problem Solving |
|
|
The day before the day after tomorrow is Monday. What is the day before the day after yesterday?
Answer Saturday.
|
|
|
Problem Solving |
|
|
Four years ago Dad was eight times as old as Junior. Today he is only four times as old. How old are they?
Answer Dad is 28, Junior is 7.
|
|
|
Problem Solving |
|
|
Kathy has eighteen pets altogether: mice, cats and budgies. The cats have twice as many legs as there are budgies and ten more legs than there are mice. How many are there of each type of pet?
Answer 6 mice, 4 cats and 8 budgies
|
|
|
Problem Solving |
|
|
LENS The problem is addition, each letter stands for a single digit. CAT is a prime number. Find the value of each letter.
Answer 1790 643 840 9297 12570
|
|
|
Problem Solving |
|
|
Find the Age Three boys were given 407 stamps, which were to be divided in proportion to their ages, the sum of which was 37 years. Brian was given five stamps for every four John received and Bill was given five stamps for every six John received. How old were the boys and how many stamps did each boy get?
Answer The boys’ ages were 15, 12 and 10 and the numbers of stamps they had were 165, 132 and 110.
|
|
|
Problem Solving |
|
|
What is the probability that a natural number less than 50 has exactly three factors?
Answer Only four of the numbers between 1 and 50 have exactly 4 factors. These are 4, 9, 25 and 49 (note all are square numbers). Therefore the probability is 4/50 = 0.08.
|
|
|
Problem Solving |
|
|
A particular month has 5 Tuesdays. The first and the last day of the month are NOT Tuesday. What is the last day of the month?
Answer The last day of the month would be a Wednesday.
|
|
|
Problem Solving |
|
|
A restaurant has a total of 30 tables which are of two types. One type seats two people and the other type seats five people. When all of the tables are full the restaurant seats 78 people. How many tables seating two are there?
Answer There would be 24 tables for two.
|
|
|
Problem Solving |
|
|
I am a 2 digit prime number, less than 60 and the sum of my digits is 7. What number am I?
Answer The number is 43.
|
|
|
Problem Solving |
|
|
Which is the most rectangular country in the world? The 'rectangularness' of a country as its maximum percentage overlap with a rectangle of the same area. (see if you can get the answer without using Google – look at a map of the world!)
Answer Egypt is the most rectangular country in the world (New Zealand was 156th) (Defined by amount of overlap of land with a rectangle.)
|
|
|
Problem Solving |
|
|
The average of n whole numbers is 80. One of the numbers is 100. After removing the number 100, the average of the remaining numbers is 78. Find the value of n.
Answer The value of n is 11.
|
|
|
Problem Solving |
|
|
How many cars? A garage has less than 50 cars. The manager arranges the cars in rows. When he puts 6 cars or 4 cars in each row, there are no cars left over. When he puts five in each row there is only one left over. How many cars does the garage have?
Answer The garage has 36 cars.
|
|
|
Problem Solving |
|
|
Paint sells for $3 a litre and paint thinner sells for $1 for 3 litres. A painter gave his helper $10 and two empty cans, telling him to bring back an equal quantity of pain and thinner and that the $10 was to cover the total cost exactly. What quantity of each item did the painter’s helper get?
Answer The helper would buy 3 litres of paint and 3 litres of thinner.
|
|
|
Problem Solving |
|
|
Anne is older than Annabel but younger than Anna. Anna is one month older than Annaliese. Annabel is two weeks younger than Anna. Who is the eldest? Arrange the girls in order of age.
Answer Anna is the oldest, followed by Anne, Annabel and Annaiese
|
|
|
Problem Solving |
|
|
Which number am I A multiple of two, that I be Not odd but even you see My digits a pair When multiplied these, make a cube and a square out of me.
Answer The number is 88
|
|
|
Problem Solving |
|
|
I’m a five digit number. All of my digits are odd. I am not divisible by 5 My 1 is neither next to 3 nor to 7 My 3 is next to 5 I neither start nor end in 9 or 1 My middle digit is not a prime number. The sum of my first two digits is half the sum of my last two.
Answer 31,597 is one solution. There are others.
|
|
|
Problem Solving |
|
|
Each letter stands for a different digit. The bottom line is the SUM of the four lines above. Find the digit represented by each letter. ABCD ABCD ABCD ABCD ------ EBEA
Answer A = 2 B = 3 C = 4 D = 8 E = 9
|
|
|
Problem Solving |
|
|
Mrs Alpa, Mrs Beta and Mrs Gamma teach English, mathematics and geography but not necessarily in that order. Each has one son. The sons’ names are Tom, Dick and Harry, again not necessarily in that order. Use the following information to work out the subject that each teacher teaches and the names of their sons.
Mrs Gamma is older than Harry. Mrs Beta and the geography teacher are twins. The mathematics teacher and Harry are the same age. Mrs Gamma is Tom’s godmother and lives next door to the geography teacher. Mrs Beta’s son is in the same class as Dick.
Answer Mrs Gamma – Geometry – Dick Mrs Beta – Mathematics – Tom Mrs Gamma - English - Harry
|
|
|
Problem Solving |
|
|
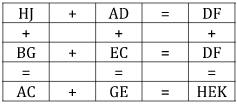
All ten digits are used in this cross-sum. What are they?
Answer A = 4, B = 2, C = 7, D = 6, E = 3, F = 5, G = 8, H = 1, K = 0, J = 9
|
|
|
Problem Solving |
|
|
Find the misfit on each line:
Answer The mis-fits were: a. 7, 17, 27, 37, 47 (27 is a composite number, the rest are prime numbers.) b. Newton, Russell, Archimedes, Cayley, Napier (Archimedes lived BC, the rest were AD) c. circle, triangle, quadrilateral, pentagon, hexagon (circle is the only non-polygons, the rest are polygons.) d. 36, 49, 81, 121, 164, 196 (164 is NOT a square number, the rest are.) e. 1, 100%, x0, 11/11, 0, 0.9999… (0.9999… is not equal to exactly 1, the rest are.) f. m2, 0.5bh, πd, 0.5(a + b)h, 2πrh (πd is the circumference of a circle, not an area, the rest are areas.)
|
|
|
Problem Solving |
|
|
A bag contains identical sized balls of different colours. 10 red, 9 white, 7 yellow, 2 blue and 1 black. Without looking into the bag, Peter takes out the balls one by one from the bag. What is the least number of balls that Peter must remove from the bag to ensure that at least three have the same colour?
Answer 10 balls must be taken out to ensure each is of the same colour.
|
|
|
Problem Solving |
|
|
An easier problem this week. Eleven years ago, why was the fifth minute after 8 o’clock in the evening on the third Tuesday in the fourth month so special?
Answer The time, date and year would all have been 2004, 2004, 2004
|
|
|
Problem Solving |
|
|
Ten years ago the ratio of John’s age to Peter’s age was 5:2. The ratio is 5:3 now. What will be the ratio in 10 years time?
Answer 10 years later the ratio will be 10:7
|
|
|
Problem Solving |
|
|
Find the next two numbers and the 20th number in the following sequences a. 19, 23, 27, … b. 3/4, 1/4, - 1/4, … c. 1, 10, 100, … d. 9, 18, 27, … e. 1, 3, 7, …
Answer a. 31, 35 and the twentieth term is 95 b. -3/4, -5/4 and the twentieth term is -8.75 c. 1000, 10,000 and the twentieth term is 1.0 x 1019 d. 36, 45 and the twentieth term is 180 e. 15, 31 and the twentieth term is 1,048,575 The King will make $110 profit.
|
|
|
Problem Solving |
|
|
The King pays $1000 for a horse. He sells it to one of his soldiers for 10% profit. The soldier later sells the horse back to the King for a 10% loss. How much profit does the King make altogether?
Answer The King will make $110 profit.
|
|
|
Problem Solving |
|
|
Mr Driver, Mr Gardener, Mr Painter and Mr Shearer are a driver, a gardener, a painter and a shearer, but none of them have a name which corresponds to their occupation. The shearer is not Mr Driver, the gardener is not Mr Painter and neither Mr Gardener nor Mr Painter is the driver. Match each man with his occupation.
Answer Mr Driver is the gardener. Mr Gardener is the painter. Mr Painter is the shearer. Mr Shearer is the driver.
|
|
|
Problem Solving |
|
|
Rights and Wrongs “How many did you get right, Viv?” “I got 5 right” replied Viv. “No, she got fewer than 5” said Kathy. “Well, I know she got more than 0” said Linda. “Actually she got more than 5 right” said Carmen. Only one person was telling the truth.
Answer Viv got none right.
|
|
|
Problem Solving |
|
|
1/7 of a group of students score an A for Mathematics, 1/3 of the students score a B, ½ score a C and the rest score a D. If a total of 100 students score an A or B, how many students score a D?
Answer 5 Score a D grade.
|
|
|
Problem Solving |
|
|
m and n are two positive whole numbers and m + n < 10 (m plus n is always less than 10). How many different values can the product mn (m multiplied by n) have?
Answer There would be 16 possible values. |
|
|
Problem Solving |
|
|
Family Dilemma In a family a boy has as many sisters as he has brothers, but each sister has only half as many sisters as brothers. How many children are there in the family?
Answer There would be 3 boys and 4 girls. |
|
|
Problem Solving |
|
|
Exponential Homework A teacher gave homework to her class and and told her students that on each day after the first, they must do twice the number of problems they had done so far. If, at the end of 5 days they had completed one third of the problems, how long will it take to do ALL of the problems?
Answer It would take 6 days to answer all the problems. |
|
|
Problem Solving |
|
|
Numbers A In how many ways can 90 be written as the product of two consecutive whole numbers?
Answer Only one 9 and 10
Numbers B In how many ways can 75 be written as the sum of at least two whole numbers all of which are consecutive?
Answer There are 3 ways: 37 and 38, 24 25 and 26, and 13 14 15 16 and 17 |
|
|
Problem Solving |
|
|
Pets 1 How many pets has Anna got if all except two are cats, all except two are dogs, and all except two are hamsters?
Answer Anna has three pets, one cat, one dog and one hamster.
Pets 2 At Alan's home there are chickens and sheep. Altogether there are 10 animals and 36 legs. How many sheep are there?
Answer Alan has three sheep and 2 chickens. |
|
|
Problem Solving |
|
|
THINK My digits they make up a pair, Whose sum and difference is a square, When multiplied they reach a score, And when pronounced they rhyme with door. What number am I?
Answer The answer is 54 |
|
|
Problem Solving |
|
|
IF If odd numbers are purple and even numbers are yellow, what colour is an odd number plus an even number? What colour is an odd number plus an odd number? What colour is an even number plus an even number? What colour is an even number plus an odd number? Answer a) Yellow |
|
|
Problem Solving |
|
|
Teacher's Age Four children were trying to guess their teacher’s age. Their guesses were 23, 28, 34 and 45 years. With a smile, the teacher told them that none of them had found his exact age. However to give them a hint, he told them that his age was between the highest and lowest of their guesses, and remarkably, the errors of their four guesses gave a total equal to his age. How old was the teacher? Answer The teacher's age is 40. |
|
|
Problem Solving |
|
|
Digital Dial-Up Jack is distraught. He can't remember Jill's telephone number. However, he does recall that all four digits are different and that the sum of the first two digits is 17. He also remembers that the sum of the middle pair of digits is 9, and that the sum of the first and last digits is 10. What number should he dial to restore his happiness? Answer The number he should dial is 8902. |
|
|
Problem Solving |
|
|
Slippery Sue When seven people asked Susie her age, her seven replies were: “I’m a teenager” “I’m not 9” “I’m 13” “I’m 12” “I’m 9” “I’m not 12” “My age is not an odd number” Susie had in fact given her age, but she had also told five lies. How old is she? Answer She is 9 years old |
|
|
Problem Solving |
|
|
Fencing Farmer Farmer Dagg planned his fence so that the posts would be exactly two metres apart but found he had five posts too few to build it this way. By placing the holes three metres apart, he had precisely the right number of posts for the fence. How long was the fence? Answer The fence is 30 metres long |
Neighbours Joe and Linda live next door to one another. The product of their house numbers is 2703. What are the house numbers? Answer The numbers were 51 and 33 |
|
Problem Solving |
|
|
Who's the tallest? Three friends, Peter, Ronald and Sandra are standing beside a stone wall. When Peter stands on Ronald's shoulders he can just see over the wall. When Ronald stands on Sandra's shoulders he does not quite reach the top of the wall. When Sandra stands on Peter's shoulders she can see over easily. Who is the tallest of the three friends? Answer Peter is the tallest |
Monkeys and Lions Answer There are 16 lions and 8 monkeys. |
|
Problem Solving |
|
|
Number Work Answer There are 8. They are 11, 13, 17, 19, 31, 41, 61, and 71 b. p and q are prime numbers. p is less than q. p + q is also a prime number. What is the value of p? Answer p = 2 and q = 3
c. I am a multiple of 6 and also a multiple of 4. The sum of my digits is a prime number. What number am I? Answer I am 12 |
Seeing the light Clue: This puzzle will have you seeing stars! Answer A star shape with lamp posts on each of the six vertices (points) and six on the hexagon in the middle. |
|
Problem Solving |
|
|
Biscuits A recipe for biscuits shows that to make 35 biscuits I will need 2 cups of flour. I want to make 210 biscuits for lunches next week. One packet of flour contains 5 cups of flour. How many packets of flour do I need? Answer You would need 2.4 packets (12 cups)
|
Jars Answer The ratio would be 17:7 |